In order to prove that entropy is a property, we will suppose two cycles i.e. 1-A-2-B-1 and 1-A-2-C-1 as shown in
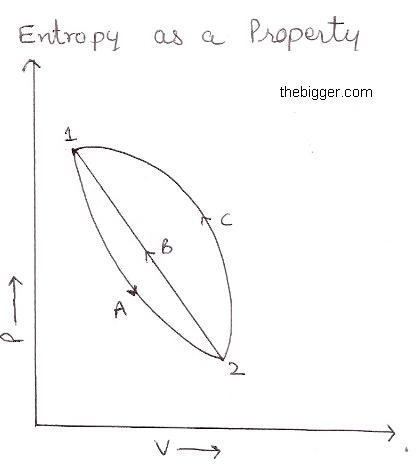
For a reversible cycle 1-A-2-B-1:
∫1-A-2 δQ / T + ∫2-B-1 δQ / T = 0
For a reversible cycle 1-A-2-C-1:
∫1-A-2 δQ / T + ∫2-C-1 δQ / T = 0
∫2-C-1 δQ / T = ∫2-B-1 δQ / T
Hence, ∫ δQ / T are a definite quantity independent of the path followed for the change and depend only upon the initial and the final states of the system. Hence entropy is a property.
